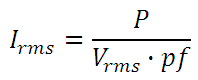In the study of electrical engineering, power factor comes up quite often in terms of its various mathematical definitions, but people seem to overlook its real-world relevance. Though there are some regulations governing power factor, the way residential users are billed for electricity often leaves us in blissful ignorance of the importance of power factor. In fact, power factor is a measure of efficiency.
Starting from first principles, let’s look at the equation for instantaneous power in electrical systems:
Of course, there are similar definitions for mechanical power (which involve torque and speed rather than voltage and current as above).
In the best case, the voltage and current waveforms will be identical, which means that they are both sinusoidal with crests and troughs occurring together. For passive devices like light bulbs (which are purely resistive loads), this is exactly what happens. However, some devices (such as capacitors and inductors) store energy for a short period of time, causing the waveform of the current to be phase shifted or displaced, relative to the voltage wave.
If we use the “average” values of voltage and current, we can determine what is known as apparent power. Even though I call them average, what we really use are the “root-mean-square” values — the reasons we use this measure are beyond the scope of this article, but suffice it to say that we can’t use a normal average for sine since it would simply be zero. For you statisticians out there, RMS is related to standard deviation (it’s a special case where your mean is zero).
Though its units are identical to those of Power (Watts), we use a different unit convention for this value, the Apparent Power (Volt-Amps, or VA).
Power factor is simply the ratio of real power compared to apparent power:
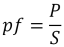For linear devices which do not store energy, real power and apparent power are the same, so the power factor is 1 (sometimes people call this “unity power factor”).
If, however, an energy storage device like an inductor or capacitor stores energy and simply return it back to the source, then the power factor will be reduced (since power is being transmitted over the line, stored temporarily and then sent back). Only real power contributes to work actually done — whether it be heating your room or turning a motor.
As mentioned earlier, inductors and capacitors cause the voltage and current to be shifted relative to each other. This results in what is called the Displacement Power Factor (the angle, φ, refers to the angular displacement between voltage and current):
However, as we are moving forward in semiconductor techologies, we are increasingly encountering more and more nonlinear devices which introduce something known as Harmonic Distortion. Basically, it makes the current waveform “noisy” compared to the voltage reference; usually this is because a device switches on and off (goes from periods of drawing some current to zero current) instead of merely being proportional to applied voltage.
Total harmonic distortion (THD) is a measure of how “noisy” the current waveform is; for example, if you draw lots of power in short-duration bursts, the current wave won’t look like a sinusoidal waveform at all. This is also known as a shape factor since it will be 1 (unity) for perfectly sinusoidal current, and smaller otherwise.
THD is measured as a percentage, so its value is somewhere between 0 and 1.
The overall power factor takes both distortion (current waveform shape) and displacement (current waveform phase difference, relative to the voltage) into account:
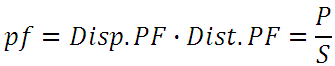So now we have identified an equation useful for determining the overall power factor of your equipment, especially for things like computers, cooking, heating, washing/drying of clothes, etc. But what does power factor have to do with efficiency?
To answer that question, let’s rearrange our power factor vs (real and apparent) power equation to look at what happens to current. We’ll be looking at RMS current, which is important because it is one of the primary factors that determines maximum loading of a given power line.
Since the amount of power we’ll need and the RMS voltage (line voltage) are effectively fixed, we can see that power factor and RMS current are inversely related. That is, with a lower power factor, we will require higher RMS current to deliver the same amount of power to our load; our RMS current is lowest when the power factor is 1 (unity).
Since power dissipated across a transmission line is:
There are finite limits on the amount of current that can be transmitted (greater power dissipated results in more heating of the wires, which can cause them to expand significantly or to melt), a unity power factor means a more effectively utilized infrastructure.
If everything we connect to the power system has a low power factor, it will result in an inefficient use of existing infrastructure (since we are transmitting more current than necessary, which also increases total line losses). It also means we will need a greater investment in infrastructure sooner, which is a challenging issue facing electric power utilities responsible for distribution of power.
Jawnsy's Journal on Life, Software and Engineering 